7.1 Long-wavelength limit

(a) At low temperatures, only the low energy (= long wavelength) modes are occupied. In the low-temperature limit, what is the equivalent of Planck's radiation law for phonons?

(b) What is the specific heat of the phonons in the low-temperature limit?

7.2 Mean number of phonons

A crystal has a volume of 1 cm³ and a sound velocity of 6000 m/s. At $T$ = 300 K, what is the number of phonons between the frequencies 4.0 × 10⁶ Hz and 4.01 × 10⁶ Hz? (Hint: 4.0 × 10⁶ Hz is much lower than the Debye frequency so the dispersion relation is linear at these frequencies and the crystal structure is not relevant. This is the long-wavelength limit. First, calculate the number of phonon modes in this frequency interval. Then use the Bose-Einstein factor to calculate the mean number of phonons in these modes.)

695

7.3 Copper

The speed of sound in copper is 3560 m/s. The crystal structure is fcc and the lattice constant (of the conventional unit cell) is $a=361.5$ pm. The point farthest from Γ on the first Brillouin zone boundary is the symmetry point W where $\overline{\Gamma W}=\sqrt{5}\pi/a$.

(a) Estimate the Debye frequency from this information.

(b) Why does the phonon dispersion relation for silver look like the phonon dispersion relation for copper? Which has a higher maximum frequency?

7.4 Phonon eigenfunctions

Show that the phonon eigenfunctions are also eigenfunctions of the translation operator. What are these eigenfunctions for a hexagonal close-packed lattice? Give the eigenfunctions in terms of $(k_x, k_y, k_z)$.

7.5 Linear chain

For a linear chain with equivalent masses connected by equivalent springs, the mass-spring system is described by the equation,

\[ \begin{equation} M\frac{d^2u_{l}}{dt^2} = C(u_{l+1}-u_l)-C(u_{l}-u_{l-1}). \end{equation} \]

Here $C$ is the effective spring constant of the bonds. Show that by substituting the general form for the normal mode solutions into this equation, the dispersion relation for a linear chain can be determined.

7.6 Linear chain with alternating spring constants

When there are more atoms in the basis, the phonon normal modes are described by a displacement vector for every atom in the basis. For two atoms in the basis, the displacement vectors are,

\[ \begin{equation} \vec{u}_{lmn} = \vec{u}_{\vec{k}}\exp\left(i\left(l\vec{k}\cdot\vec{a}_1+m\vec{k}\cdot\vec{a}_2+n\vec{k}\cdot\vec{a}_3-\omega t\right)\right), \end{equation} \] \[ \begin{equation} \vec{v}_{lmn} = \vec{v}_{\vec{k}}\exp\left(i\left(l\vec{k}\cdot\vec{a}_1+m\vec{k}\cdot\vec{a}_2+n\vec{k}\cdot\vec{a}_3-\omega t\right)\right). \end{equation} \]

Consider a linear chain of equilavent masses but alternating spring constants $C_1$ and $C_2$.

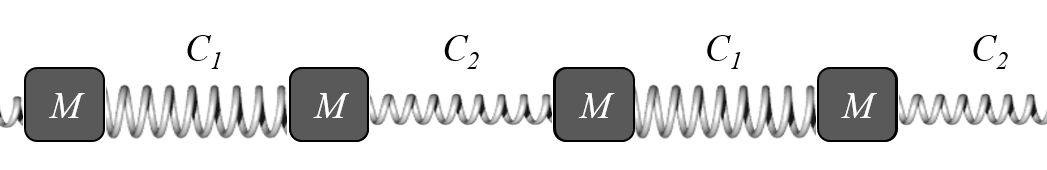
If the atoms are confined to motion in one-dimension the displacements are,

$$u_{l} = u_{k}\exp\left(i\left(lka-\omega t\right)\right),\qquad v_{l} = v_{k}\exp\left(i\left(lka-\omega t\right)\right).$$

What are the equations of motion that describe this system? Show that by substituting the general form for the normal mode solutions into this equation, the dispersion relation can be determined.

This problem is similar to a 1-d chain of atoms with two different masses.

Thermodynamic properties

Many thermodynamic properties can be calculated from the density of states. Since phonons are bosons, the formula's are:

Internal energy density:	\begin{equation} u = \int \limits_{0}^{\infty} \frac{\hbar\omega D(\omega)}{\exp\left( \frac{\hbar\omega}{k_BT} \right)-1}d\omega \end{equation}
Specific heat:	\begin{equation}c_v=\frac{\partial u}{\partial T}=\int \limits_{0}^{\infty}\frac{\hbar^2\omega^2 D(\omega)\exp\left(\frac{\hbar\omega}{k_BT}\right)}{k_BT^2\left[\exp\left(\frac{\hbar\omega}{k_BT}\right)-1\right]^2}d\omega \end{equation}
Entropy density:	\begin{equation} s = \int \frac{c_v}{T}dT =-k_B \int \limits_{0}^{\infty}D(\omega)\left( \ln \left[ 1-\exp\left( \frac{-\hbar\omega}{k_BT} \right) \right]+\frac{\hbar\omega}{k_BT\left(1-\exp\left( \frac{\hbar\omega}{k_BT} \right)\right) } \right) d\omega \end{equation}
Helmholz free energy density:	\begin{equation} f=u-Ts= \int \limits_{0}^{\infty} D(\omega) \left( k_BT\ln\left[ 1-\exp\left( \frac{-\hbar\omega}{k_BT} \right)\right] \right) dE \end{equation}

7.7 Thermodynamic properties

(a) The integrals that have to be performed to calculate the phonon contribution of thermodynamic properties such as the internal energy or Helmholtz free energy only need to be integrated over a finite frequency range. Why is this?

(b) The Debye temperature $T_D$ is defined by, $k_BT_D = \hbar \omega_D$, where $\omega_D$ is the frequency of the highest phonon mode. For $T > > T_D$ show that the internal energy density,

$$u=\int\limits_0^{\omega_D}\hbar\omega D(\omega)f_{BE}(\omega) d\omega$$

is proportional to the temperature $T$. Here $D(\omega)$ is the phonon density of states and $f_{BE}(\omega)$ is the Bose-Einstein factor. A consequence of this is that the phonon contribution to the specific heat is constant for $T > > T_D$.

(c) Calculate the specific heat and the derivative of the specific heat with respect to temperature $dc_v/dT$ of silicon at 300 K. Use this table of the phonon density of states and this program to calculate the specific heat.

(c) The program gives $c_v=$ 1624329 [J K^-1 m^-3] and $dc_v/dT=$ 2387 [J K^-2 m^-3].

7.8 Phonon Dispersion

Below is the phonon dispersion relation for a crystal with an fcc Bravais lattice. The lattice constant is a = 0.2 nm.

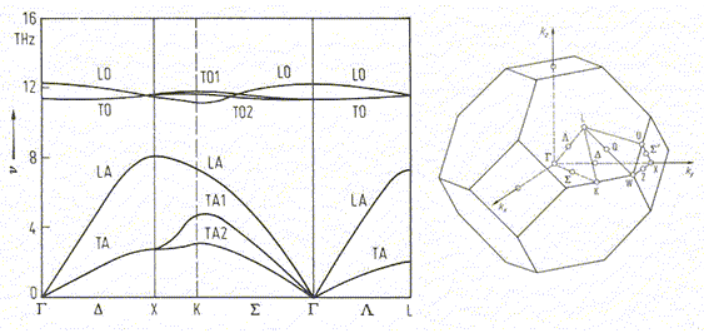
(a) How many atoms are there in the primitive unit cell?

(b) Choose a direction and a polarization (longitudinal or transverse) and estimate the speed of sound in this direction for long-wavelength sound waves.

(c) What is the shortest phonon wavelength possible in this crystal?

(d) The Bose-Einstein factor tells us the mean number of bosons in a state of energy $\hbar\omega$ at temperature $T$. This number can be greater than or less than 1. What is the energy of a phonon state with a mean occupation of 0.4 bosons at 300 K?

Hint: Note that the dispersion plot uses ν in THz (instead of ω)

7.9 Diamond

Diamond has an fcc Bravais lattice. The primitive lattice vectors are,

$\vec{a}_1=\frac{a}{2}\hat{x}+\frac{a}{2}\hat{y}$, $\vec{a}_2=\frac{a}{2}\hat{x}+\frac{a}{2}\hat{z}$, $\vec{a}_3=\frac{a}{2}\hat{y}+\frac{a}{2}\hat{z}$.

There are two atoms in the basis. The positions of the two carbon atoms given in terms of the fractional coordinates of the conventional unit are,

$\vec{B}_1=(0,0,0)$, $\vec{B}_2=(0.25,0.25,0.25)$.

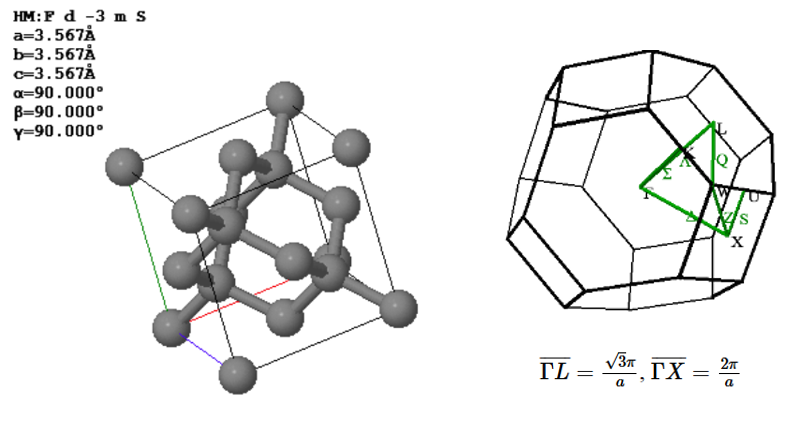
(a) How many $\vec{k}$ vectors satisfy periodic boundary conditions for a diamond crystal 1 cm³? (The lattice constant is $a=3.567$ Å.)

(b) The lattice vibrations can be described in terms of normal modes. How many normal modes does a diamond crystal 1 cm³ have?

(c) Use the empty lattice approximation to sketch approximately the phonon dispersion relation for diamond along $L-\Gamma -X$.

7.10 Phonons in aluminum

In aluminum, the longitudinal sound velocity is $c_l=6.32\times 10^3$ m/s and the transverse sound velocity is $c_t=3.1\times 10^3$ m/s. The density of Al is $2.7\times 10^3$ kg/m³, and its atomic weight is 26.97 u. In the Debye model, the phonon dispersion relation is linear up until a maximum frequency $\omega_D$. However, for many materials like aluminum, there are two sound speeds. Divide the normal modes into the 1/3 that are longitudinal and the 2/3 that are transverse and construct both densities of states using the assumptions of the Debye model.

(a) The total number of normal modes is finite. Calculate the frequency of the phonon mode with the highest frequency.

(b) The Debye temperature for aluminum, as obtained from specific heat measurement is 375 K. Determine the Debye frequency from this measurement and compare it with the result from (a).

(c) At low temperatures, the density of states has the form $D(\omega ) = \alpha\omega^2$. Determine the low-temperature form of the phonon contribution to the specific heat of aluminum. It may be useful to know that,

$$\int_0 ^\infty \frac{x^3}{e^x - 1} \mathrm{d}x = \frac{\pi^4}{15}.$$

7.11 Effective spring constant

The highest frequency phonon mode of an fcc lattice is $2\sqrt{\frac{2C}{m}}$ where $C$ is the effective spring constant. Use a calculated density of states to determine the effective spring constant for Al, Cu, Ag, and Pb?

7.12 Empty lattice approximation

Use the empty lattice approximation to sketch the phonon dispersion relation for Cr (bcc), NaCl (fcc), and SrTiO₃ (simple cubic).

7.13 CsI

CsI has a simple cubic Bravais lattice with a Cs atom at (0,0,0) and an I atom at (0.5 0.5 0.5). Which of the figures below is the phonon dispersion curve for CsI? Explain your reasoning.

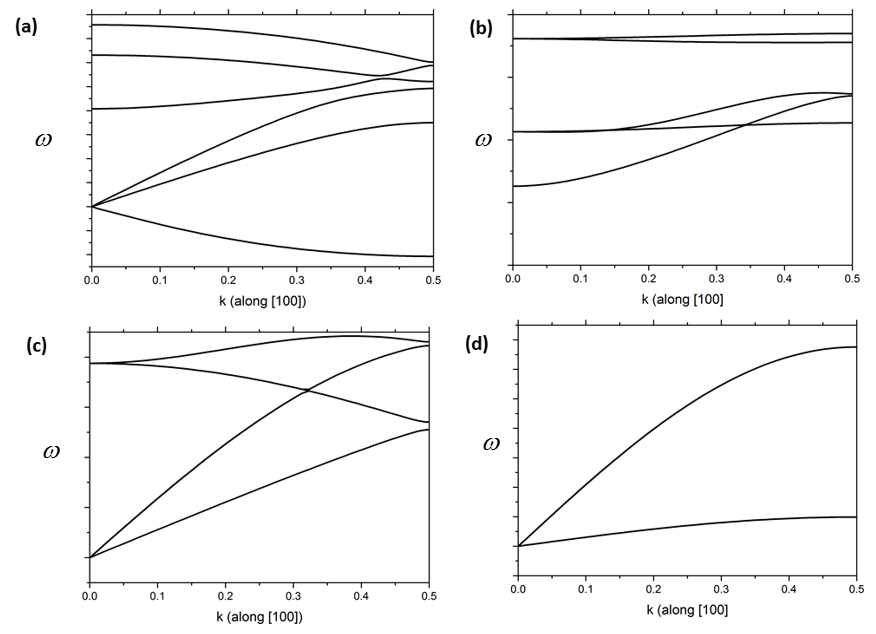
7.14 Density of states

(a) If the phonon dispersion relation is known, describe how to calculate the phonon density of states.

(b) The ratio of the area under the phonon density of states of the optical modes divided by the area under the phonon density of states of the acoustic modes is always an integer. What determines this integer?

(c) Calculate the density of silver from the phonon density of states. By numerically integrating over all frequencies it is possible to determine the number of phonon modes per m³. This must be equal to the number of vibrational degrees of freedom per m³. The number of vibrational degrees of freedom is 3 times the number of atoms per m³. Calculate the concentration of atoms per m³ and multiply by the mass of an atom to get the density.

7.15 Diamond (2)

A crystal has the diamond structure which has an fcc Bravais lattice. The primitive lattice vectors are,

$\vec{a}_1=\frac{a}{2}\hat{x}+\frac{a}{2}\hat{y}$, $\vec{a}_2=\frac{a}{2}\hat{x}+\frac{a}{2}\hat{z}$, $\vec{a}_3=\frac{a}{2}\hat{y}+\frac{a}{2}\hat{z}$,

where $a=3$ Å. There are two atoms in the basis. The positions of the two atoms given in terms of the fractional coordinates of the conventional unit are,

$\vec{B}_1=(0,0,0)$, $\vec{B}_2=(0.25,0.25,0.25)$.

(a) How many $\vec{k}$ vectors satisfy periodic boundary conditions for 7 cm³ of this crystal?

7.16 Displacement of an atom in a normal mode

A phonon normal mode has a frequency of $\omega = 10^{12}$ rad/s. The displacement of an atom is described by,

Where is this atom at time $t=$ 7 ps?

7.17 The number of k states in the first Brillouin zone

Show that there are as many translational symmetries as there are Bravais lattice points in a crystal and show there are as many reciprocal lattice points in the first Brillouin zone as there are Bravais lattice points in a crystal.

7.18 GaN

The phonon dispersion relation of GaN is shown below.

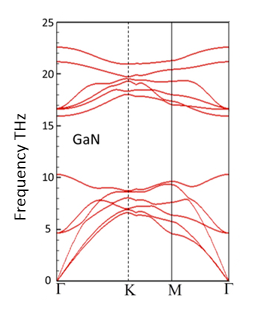
(a) How many atoms are there in the basis of GaN?

(b) Write down the formula for the normal modes of GaN.

(c) Estimate the frequency and the wavelength of the optical mode with the lowest frequency at the Γ point from the plot.

(d) What is the density of occupied phonon states in a frequency interval $\Delta \omega=10^4$ [rad/s] centered at an angular frequency of $50 \times 10^{12}$ rad/s at 300 K?

(d) The number of occupied states in this interval is $7.777\times 10^{18}$ m^-3.

7.19 Specific heat of GaN

What is the phonon contribution to the specific heat of GaN at $T=300$ K?

Use the phonon density for GaN.

Phonons

Low-frequency (long-wavelength) limit