Name Matrikelnr.
Every subproblem (a), (b), (c), etc. is worth 5 points.
Problem 1
A methyl radical consists of one carbon atom and three hydrogen atoms. Carbon has six protons and hydrogen has one proton. Radicals are electrically neutral.
(a) Solutions to the molecular orbital Hamiltonian for a methyl radical are sought using a trial wave function that includes the carbon 2s and carbon 2p orbitals as well as the hydrogen 1s orbitals. How many molecular orbitals will be found?
(b) How will the molecular orbitals found in (a) be occupied in the ground state of the methyl radical?
(c) A Slater determinant is constructed out of the molecular orbitals found in (a) to describe the many electron wave function of the ground state. This is the determinant of an $N\times N$ matrix. What is $N$?
(d) If two columns of the Slater determinant are exchanged, what happens to the wave function? Why?
(e) How many vibrational and rotational modes does the methyl radical have?
(f) Are the rotational energy levels of a methyl radical more closely spaced or more widely spaced that the rotational states of a H2 molecule? Why?
Problem 2
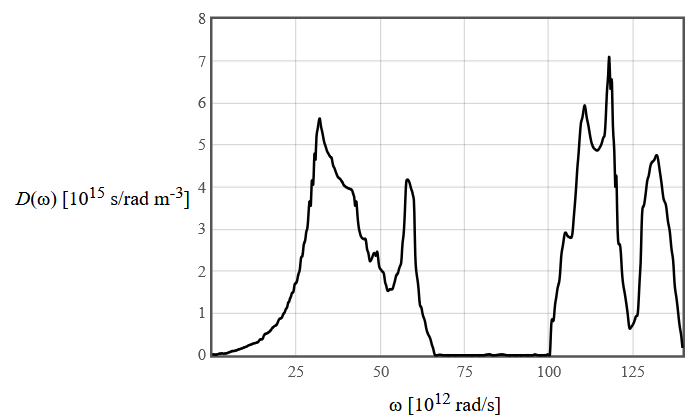
A phonon density of states is shown below. The modes below $75\times 10^{12}$ rad/s are the acoustic modes.

(a) How many atoms are there in the primitive unit cell? Explain how you know this.
(b) What is the mean number of phonons in the optical mode with the lowest frequency at 300 K?
(c) Express the phonon contribution to the specific heat as an integral including the phonon density of states.
Problem 3
The electronic band structure for a SiC in a zincblende (fcc) crystal structure is shown below. The lattice constant is $a = 4.36$ Å. The zero of energy is the top of the valence band.
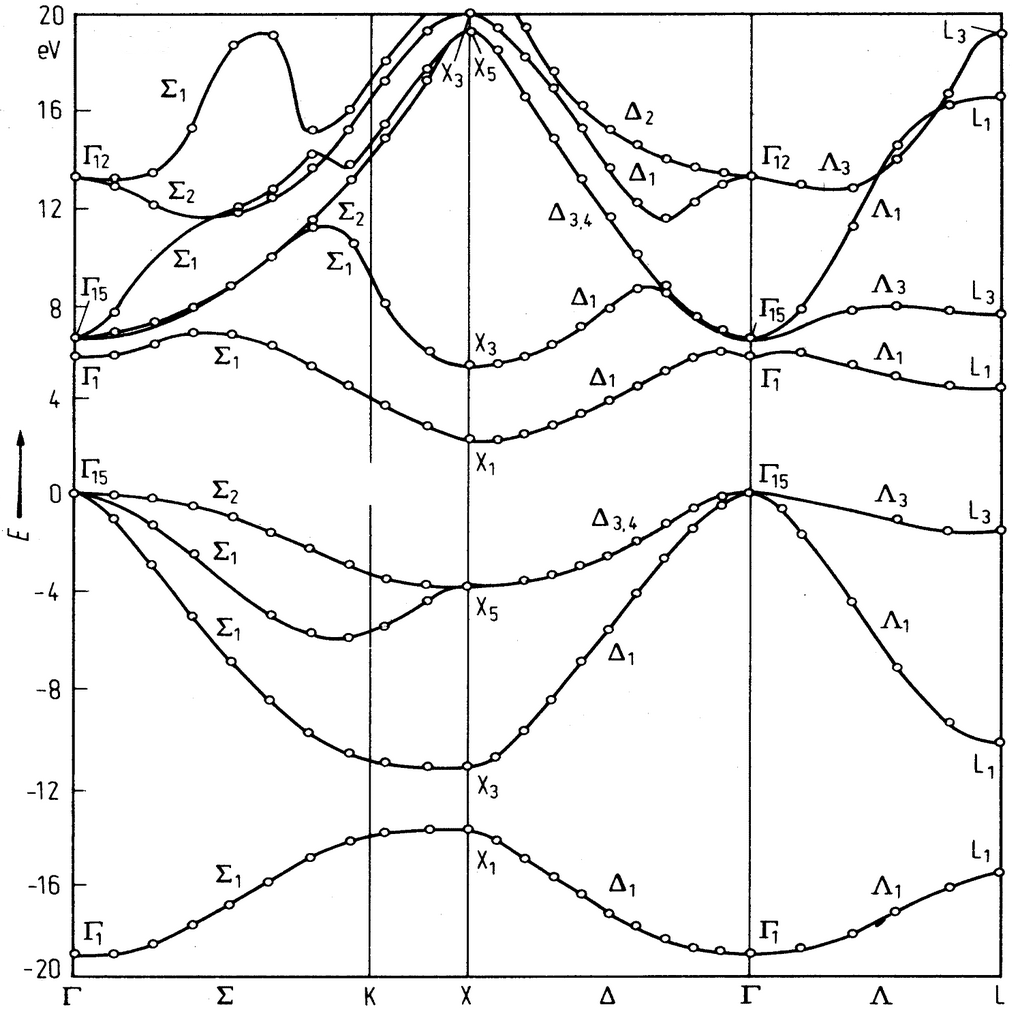
(a) Indicate the band gap in the band structure diagram. Draw an arrow to show where the light holes are.
(b) Is this a direct or an indirect band gap semiconductor?
(c) How many valence band maxima and conduction band minima are there? This can be deduced from the symmetry of the crystal.
(d) Express the concentration of holes in the valence band as an integral over the density of states.
(e) Four valence bands are shown. How many electrons per primitive unit cell are needed to fill these bands? Explain how you know this.
Problem 4
A simple cubic crystal with one atom in the basis has a lattice constant of π Å. The atomic form factor for the atom is $f(G)=84\exp\left( -0.05G^2\right)$ where $G$ is measured in Å-1.
(a) What is the longest wavelength that can be diffracted by this crystal?
(b) The intensities of the diffraction peaks are proportional to the square of the structure factors. What is the structure factor for $G_{111}$?
(c) X-rays with a wavelength of 0.3 Å are used to measure this material in a powder diffraction experiment. At which angle will the reflection appear for $G_{100}$?