PHY.F20 Molecular and Solid State Physics
10.05.2016

Problem 1

The molecule 2-ethenyl-1,3 butadien is an aromatic molecule, the chemical structure is depicted below.

a) The carbon atoms in this molecule form sp² σ-bonds with their neighbors. The valence orbitals are the carbon 2p_z orbitals which form π-bonds. How many electrons are there in this molecule and how many participate in π-bonds?

b) We now restrict our attention to the valence electrons. Use a linear combination of atomic orbitals to write down a wave function that describes the electrons that participate in the π-bonds. Use the notation that the position of atom C₁ is $\vec{r}_1$.

c) Write down the form of the Roothaan equations for this molecule including the Hamiltonian matrix and the overlap matrix.

d) In Hückel theory, the form of both the Hamiltonian matrix and the overlap matrix are simplified. Please write down the simplified Hamilton matrix and overlap matrix. What are the simplifications and why are they justified?

Solution

<hr />
(a) Total number of electrons in molecule: Carbon: 6*6 = 36 + Hydrogen: 1*8 = 8 = 44 electrons.

Carbon has six electrons. Two of the electrons of each carbon atom fill the 1s orbital. We are told that the carbon atoms form sp² bonds. Three electrons of each carbon atom form the sp² bonds. This leaves one electron per carbon atom in the 2pz orbital. In the molecule there are six valence electrons in the 2pz orbitals that participate in the π-bonds.

(b) The trial wave function consisting of the 6 2pz orbitals is,

$$\psi(\vec{r}) = \sum_{i=1}^6 c_i \phi_{2pz}^{Z=6}(\vec{r}-\vec{r}_i).$$

Here $c_i$ are coefficients that will be determined by solving the Roothaan equations.

(c) Roothaan equations consist of a 6 × 6 Hamiltonian matrix $\textbf{H}$, and a 6 × 6 overlap matrix $\textbf{S}$,

$$\textbf{H}\,\vec{c}=E\textbf{S}\,\vec{c}, $$

where $E$ is the energy of the molecular orbitals and,

$$ H_{pq}= \langle\phi_{p}|H_{\text{mo}}|\phi_{q}\rangle \hspace{1cm}\text{and}\hspace{1cm}  S_{pq}= \langle\phi_{p}|\phi_{q}\rangle .$$

(d) In the Hückel model, all of the Hamiltonian matrix elements that do not correspond to on-site or nearest neighbor interactions are set to zero and the overlap matrix is taken to be the identity matrix. In this case, the Roothaan equations take the form,
$$\left( 
 \begin{array}{cccccc}
 H_{11}&H_{12}&0&0& 0& 0\\
 H_{21}&H_{22}&H_{23}&0 & 0 & 0\\
 0&H_{32}&H_{33} &H_{34}&H_{35}&0\\
 0&0&H_{43}&H_{44}&0&0\\
 0&0&H_{53}&0&H_{55}&H_{56}\\
 0&0&0&0&H_{65}&H_{66} 
 \end{array}
 \right) 
 \left(\begin{array}{c}
 c_1\\
 c_2\\
 c_3\\
 c_4\\
 c_5\\
 c_6\end{array}\right) = E\,
 \left(\begin{array}{c}
 c_1\\
 c_2\\
 c_3\\
 c_4\\
 c_5\\
 c_6\end{array}\right)$$

These approximations can be made since the atomic orbitals decay exponentially with distance so that the matrix elements of atoms further away than nearest neighbors are very small. 
Roothaan and Hückel solved these equations by hand. Using modern computers, there is no need to use the Hückel approximation anymore.

Problem 2

CaTiO₃ has what is known as the ideal perovskite structure. Calcium atoms are at the corners of cube, a titanium atom is at cube body center, and oxygen atoms are at cube face centers. Take the cube edge length to be $a$.

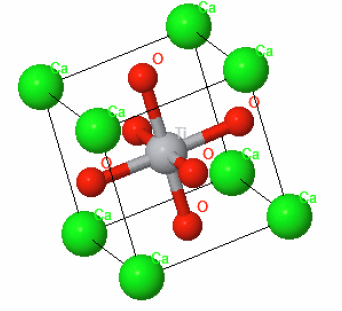
a) What is the Bravais lattice type?

b) Write down a set of primitive lattice vectors.

c) Verify that there are 3 oxygen atoms, 1 titanium atom and 1 calcium atom the basis of this crystal structure.

d) Give the positions of the atoms in the basis in fractional coordinates of the primitive lattice vectors.

Solution

Problem 3

A diffraction experiment is performed on a crystal with a primitive cubic lattice with a lattice constant of $a = 3$ Å. The electron density can be approximated by point charges $10e$ at the corners of the cube and $5e$ at the body center.

a) Calculate the primitive lattice vectors of the reciprocal space lattice.

b) Calculate the length of the reciprocal lattice vector 124 $(h =1,\, k = 2,\, l =4)$. Give the units in your answer.

c) At which scattering angle 2θ is the diffraction of the 124 reflection is observed, if the wavelength of the X-rays is 1.1 Å?

d) Calculate the structure factor of the 124 reflection.

Solution

<hr />
(a) The reciprocal lattice vectors of a simple cubic lattice with lattice constant $a=3$ Å are,

$$\vec{b}_1 = 2\pi \frac{(\vec{a}_2\times \vec{a}_3)}{\vec{a}_1(\vec{a}_2\times \vec{a}_3)} = \frac{2\pi}{a} \begin{bmatrix} 1\\ 0 \\ 0 \end{bmatrix}, \\ 
\vec{b}_2 = 2\pi \frac{(\vec{a}_3\times \vec{a}_1)}{\vec{a}_1(\vec{a}_2\times \vec{a}_3)} = \frac{2\pi}{a} \begin{bmatrix} 0\\ 1 \\ 0 \end{bmatrix}, \\ 
\vec{b}_3 = 2\pi \frac{(\vec{a}_1\times \vec{a}_2)}{\vec{a}_1(\vec{a}_2\times \vec{a}_3)} = \frac{2\pi}{a} \begin{bmatrix} 0\\ 0 \\ 1 \end{bmatrix}.$$

(b) The reciprocal lattice vector $\vec{G}_{124} = \vec{b}_1 + 2\vec{b}_2 + 4\vec{b}_3$. The length of this vector is 
$|\vec{G}_{124}| = \frac{2\pi}{3}\sqrt{1^2+2^2+4^2}$ Å$^{-1}$ = 9.60 Å$^{-1}$.

(c) The diffraction condition is $\Delta\vec{k}=\vec{G}$ and $2\theta$ is the angle between $\vec{k}$ and $\vec{k}'$. Since the condition for elastic scattering $|\vec{k}|=|\vec{k}'|$ is satisfied, we can construct a right triangle where,

$$\sin(\theta) = \frac{\frac{|G_{124}|}{2}}{|\vec{k}|}$$

Using the definition of wavenumber, $|\vec{k}| = \frac{2\pi}{\lambda}$, $\theta$ can then be computed to be, 
$\theta = 57.2$°,   $2\theta = 114.3$°.

(d) The structure factor for a point charge distribution is,

\begin{equation}
 S_{\vec{G}}=\sum \limits_j Z_j\exp (-i\vec{G}\cdot\vec{r}_j),
\end{equation}

The structure factor $S_{124}$ is,

$$S_{124}=10+5\exp \left(-i\left(\vec{b}_1\cdot\frac{\vec{a}_1}{2}+2\vec{b}_2\cdot\frac{\vec{a}_2}{2}+4\vec{b}_3\cdot\frac{\vec{a}_3}{2}\right)\right)=10 +5\exp \left(-i(\pi+2\pi+4\pi)\right)=5.$$

PHY.F20 Molecular and Solid State Physics10.05.2016

PHY.F20 Molecular and Solid State Physics
10.05.2016